Dr. Ian Malcolm - Jeffrey Lynn Goldblum
Unlike the other scientists Dr. Ian Malcolm was a mathematician who has devoted his life in the research of the “Chaos Theory”. Interestingly, the quotes by Dr. Ian Malcolm “Life, uh, finds its way” is one of the most important quote and thesis of the whole Jurassic Park and Jurassic World series.
Dr. Ian Malcolm was invited to Jurassic Park by the Donald Gennaro a lawyer hired by the investors who were concerned of the death of the worker Jophery Brown by the velociraptors. Ian Malcolm was skeptical of Ingen’s capability to control ‘life’ of the dinosaurs.




Ian Malcolm explains his concerns in a short and brief sentence that "Life finds a way". Ian Malcolm was sent to a hospital in Costa Rica after the successful escape from Jurassic Park where his girl friend Sarah Harding was searching for him.
Ian Malcolm despite having a confidentiality agreement publishes a book of the Isla Nublar Jurassic Park incident where Ingen successfully manipulated the media and made a fool out of Dr. Ian Malcom.
Jeffrey Lynn Goldblum is an actor and musician who has played the role as Dr. Ian Malcolm. Jeffrey Lynn Goldblum has starred in many films such as Independence Day as a satellite engineer David Levinson, and the Grand Master in the Marvel Cinematic series.




Dr. Ian Malcolm’s experiment with water with Dr. Ellie Sattler explained
Dr. Ian Malcolm kind of uses a glass of water to flirt with Dr. Ellie Sattler. However, he demonstrates an example of the Chaos Theory by drops of water on Dr. Ellie Sattler’s hands.
Dr. Ian Malcolm simply asks Dr. Ellie Sattler to predict where a water drop would fall over from her hands after he dropped a single drop of water. Dr. Ellie Sattler predicts that the next drop of water shall also fall into the same direction as the first drop of water did.
Dr Ellie Sattler makes this prediction because Dr. Ian Malcolm never relocated the position of the water drop and Dr. Ellie Sattler never relocated her hand as well.



However, after Dr. Ian Malcolm drops another water drop on the same position on the same location on Dr. Ellie Sattler’s hands, the water drops into a totally different direction.
Dr. Ian Malcolm explains that the after the first water drop, the slightest changes of Ellie Sattler’s hands such as the shivering from the water drop, the retraction of the blood vessels, the shrinking of the skin pores etc has in fact greatly changed the “initial conditions” of her hand leading to the next water drop to fall into a totally different direction.
By this experiment, Dr. Ian Malcolm wanted to explain that by the Chaos Theory, even in a simple system that consists of only a human hand with a drop of water from a single glass of water, the direction of the water drop on a human’s hand cannot be predicted nor controlled.




Why is Dr. Ian Malcolm, a mathematician present such a significant thesis of Jurassic Park
Dr. Ian Malcolm unlike Dr. Ellie Sattler who is a paleobotanists that studies ancient herbology and plants and paleontologist Dr. Grant who studies ancient animals(dinosaurs), is a mathematician, but presents and holds the most important thesis of Jurassic Park depicted by his famous lines “Life, uh, finds its ways”.
Unlike the most traditional debates topics over the creation of life, ethics and dinosaurs between theologists and existential science, the whole theme of Jurassic Park doesn’t focus much on the ethics of creation or the arrogance of playing God although there are some events and consequences of such themes throughout the whole series.



Jurassic Park whether focuses on the delivery of thesis that “Nature is never controllable. Life and the world shall always exist beyond our comprehension”.
This thesis has been the major premise of the whole Jurassic Park and Jurassic World series while there were many other themes and topics that the audience could think through.
Not a single plan or event of the Jurassic Park and Jurassic World series whether well-orchestrated or not has ever been well executed or proceeded as plan and all the characters had to act through the uncertainty. Dr. Ian Malcolm has asserted that even the smallest repetitive events cannot be predicted nor controllable by the Chaos Theory.


He presented that events that himself as a human throughout life and the whole creation of Jurassic Park would be full of unprecedented consequences.
Thus, “Life finds its way” not only refers that Jurassic Park cannot control all the dinosaurs, but it also refers that as an ordinary human being, every character shall also have to face challenges and navigate through the unpredictable aspects of life.
Dr. Ian Malcolm and his Chaos Theory was one of the most perfect deliveries of the whole thesis that points throughout the whole Jurassic Park and Jurassic World series.



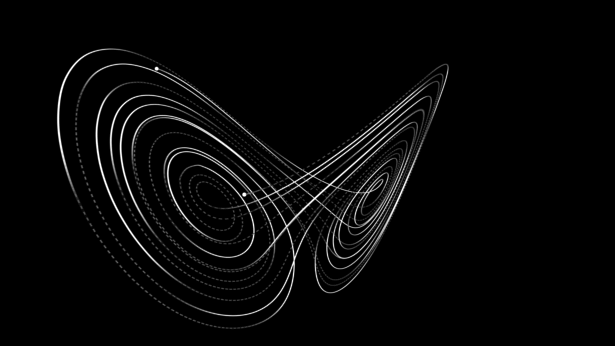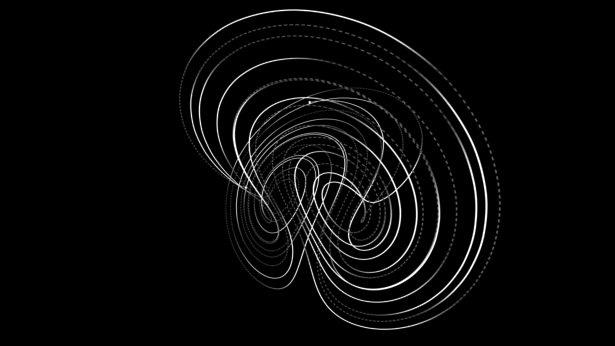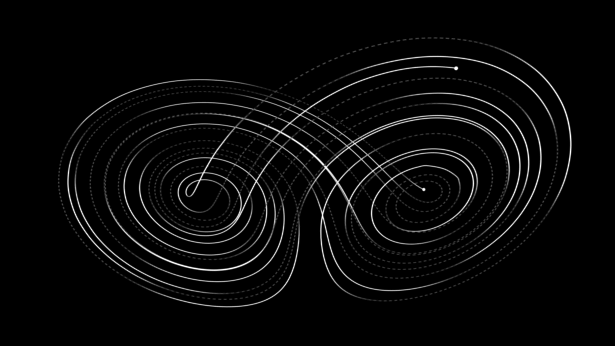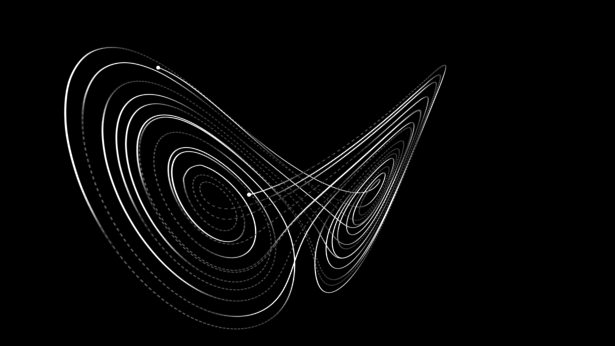
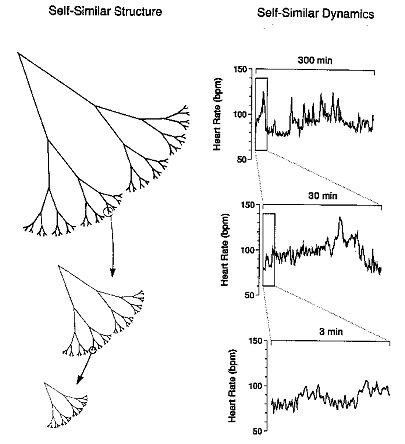
The Chaos Theory
The Chaos Theory is a mathematic concept that is also called “Non-Linear Dynamics” A nonlinear system is a system where the result of an input of a value isn’t proportional.
Although the Chaos Theory is most famous as the “Butterfly Effect” that states a single flap of a butterfly’s wing could cause a hurricane, the Chaos Theory doesn’t necessarily infer that a small variable could cause such a huge consequence.
The Chaos Theory rather focuses on the function or relationship of a system as below that shows a chaotic theory.
The Chaos Theory also implies that despite a random pattern, by careful examination of the initial values and relationship of the input and the outcome, a relationship could exist.

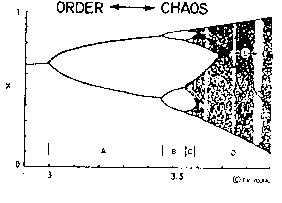
If the universe is simplified as a chaotic dynamic system, it is impossible to set up the initial settings of nature, thus it is impossible to predict the future according to the Chaos Theory.
The Chaos Theory doesn’t necessarily exploit that the future is not predictable but if a system is a chaotic dynamic system, that incorporating all the initial variables and value is required for prediction.
Thus Dr. Ian Malcolm warns John Hammond and InGen of the unpredictable consequences of playing God by reviving the dinosaurs. Dr. Ian Malcolm asserts the uncertainty by the line that “God creates dinosaurs, God kills dinosaurs, God creates man, man kills God, man brings back dinosaurs.”
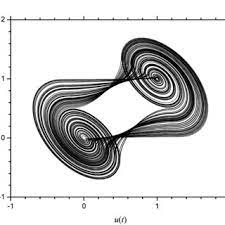

Conditions of the Chaos Theory/Chaotic Dynamic System
1. The system should be sensitive to the Initial set up
2. The system should show Topological mixing
The definition of Topological Mixing refers to a system that could define any kind of mixing within the topology of the system.
In other words, if any kind of random pair of set is selected within the system and there is a value iteration within the system, this behavior is called Topological mixing.
3. The system should have dense periodic orbit
An orbit refers to the set of outcomes that derive from initial input after time has passed. A periodic orbit refers that after a certain amount of time, the orbit would return to the initial input.
A Dense periodic orbit refers to the fact that the union of the periodic orbit would form a dense set that corresponds to all of the initial input.
Easy example of the Chaos Theory – Pin ball
The example of a chaos theory could be a pinball. The box where the pinball is located is a dense periodic orbit as the box that surrounds the pinball is the union of all the possible locations that the pinball could be located.
Any kind of location within the box, the pinball could be located by chance, thus the pinball box shows topological mixing.
Although the pinball is bounced off by the same power, speed, the pinball would bounce off to a different location every time, thus the bouncing of the pinball by a pin is extremely sensitive to the initial set up.
Even bouncing a simple pinball would never have the same result, and this serves as a good example to explain the chaos theory.



Early ideas of the Chaos Theory - Henri Poincaré
Henri Poincaré a French mathematician and theoretical physicist has made an argument on the claims that the history of the solar system could be calculated where the calculations could be used to predict the future of the solar system.
Researchers have claimed that if the first state of the solar system is discovered that they could predict the future of the solar system.
However, Henri Poincaré disagreed and claimed “Sensitivity to Initial Conditions” exist and claimed that no matter how precise, researchers could understand the nature and state of the solar system, even the slightest differences in the initial conditions or a simple preclusion of an unknown factor could lead to a massive error.
Henri Poincaré’s concept of “Sensitivity to Initial Conditions” would later lead to the development of the Chaos.


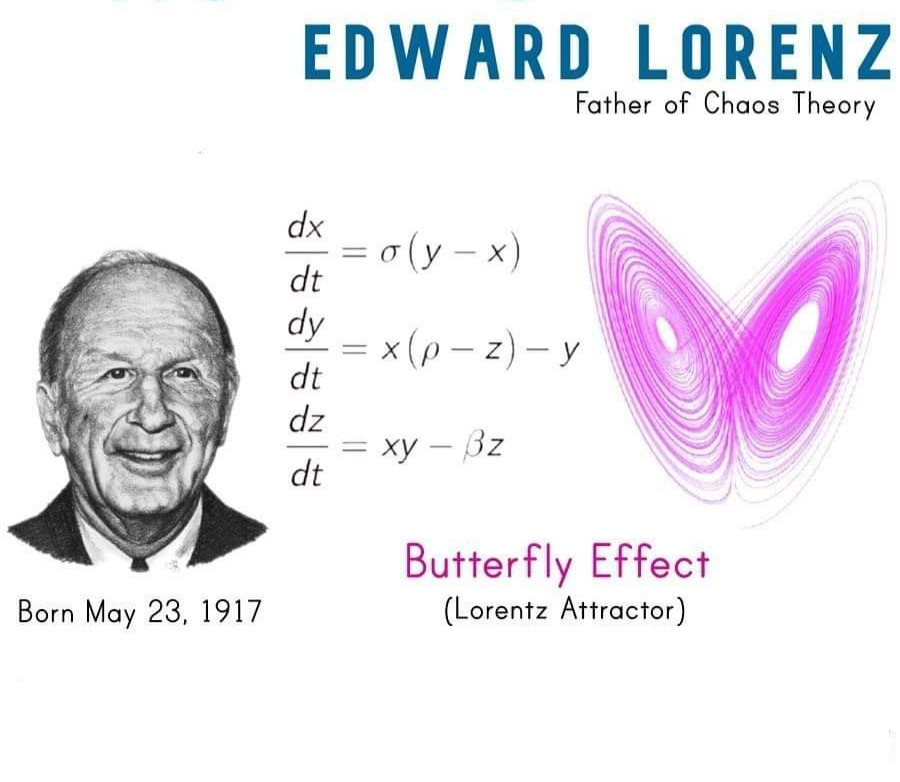
Birth of the Chaos Theory – Edward Lorenz
Edward Norton Lorenze who was a meteorologist and mathematician from Massachusetts Institute of Technology (MIT) has discovered that despite using the same weather prediction model, when he used a value to the 3rd decimal and a value to the 6th decimal, the outcomes of the model were completely different, thus the weather prediction was totally different.



At a conference, Edward Lorenz addressed this phenomenon as the chaotic behavior of a nonlinear system, however the title of this speech by the organizer of the conference referred this as the famous : “Predictability: does the flap of a butterfly's wing in Brazil set off a tornado in Texas?” and later on Edward Lorenz’s theory became the Chaos Theory.


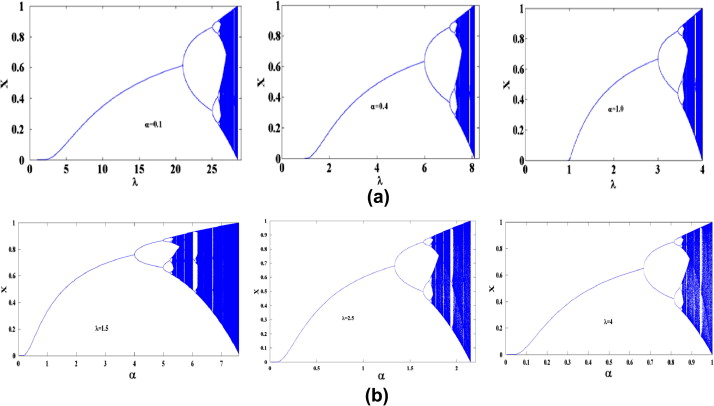
Logistic map – Derivative of the Chaos Theory
The Logistic Map is a function that has derived from the Chaos Theory developed by a biologist named Robert M. May who simply shows that a simple two-degree function could show chaotic behavior, in other words depending on the number and possible range, the outcome of a function could vastly vary.



Where xn stands for a random possibility which is a number between 0 and 1, depending on the range of r, the outcome of the function, would fluctuate showing that even for such a simple function, the outcome is very sensitive to the initial conditions set. The Logistic Map shows how such a simple function could show a chaotic behavior by a graph easily.
xn+1=rxn(1-xn)